Edit: This activity follows a broadly constructivist approach. Since running this activity last year, and in light of the evidence, my view on constructivist teaching methods has changed. If I were to use this activity again, I’d change the order. That is, I wouldn’t run it as a way to try to get students to discover the embedded concepts, instead I’d run it as a way to consolidate the knowledge of concepts previously taught via direct instruction. I leave old posts like this on my blog because I think it’s valuable to have a record of how my views and approaches to teaching have changed over time, and continue to change.
At the recent Educational Changemakers 2016 conference (EC16) I came across the work of Shane Loader and the Empowering local learners project. After an engaging chat at the post-conference drinks, Shane was generous enough to share with me some of the top blogs that he follows as well as a link to the Empowering Local Learners (ELL) project that he’s currently working on. Whilst browsing the site I found a great lesson plan on surface area and volume that gave me an idea for my Methods Class. The activity: ‘What’s my function?’.
The previous few lessons had been on introducing the derivative function. Students were able to calculate the derivative of a polynomial both from first principals and ‘the rule’. The goal of this lesson was to scaffold students to make the link between the zeroes of a derivative function and the turning points of its parent/primitive function.
I split my (luxuriously small) class into two groups and gave each group one of the following instruction sheets.
 Students were excited! They were straight onto Desmos to explore their functions
Students were excited! They were straight onto Desmos to explore their functions
Then they jumped into asking their questions. Here’s how the discussion went…
 The students were taking the activity really seriously, and being very competitive. Due their deep consideration of the questions they were going to ask, they were really taking their time, and we were fast coming up to the end of the period. With about 15-20 minutes to go I took advantage of my teacher privilege with the following change to the rules (I recorded the following in class).
The students were taking the activity really seriously, and being very competitive. Due their deep consideration of the questions they were going to ask, they were really taking their time, and we were fast coming up to the end of the period. With about 15-20 minutes to go I took advantage of my teacher privilege with the following change to the rules (I recorded the following in class).
Here’s he chat that followed… (The team on the left knew their original fn was a cubic, and the other knew they were looking for a quartic).
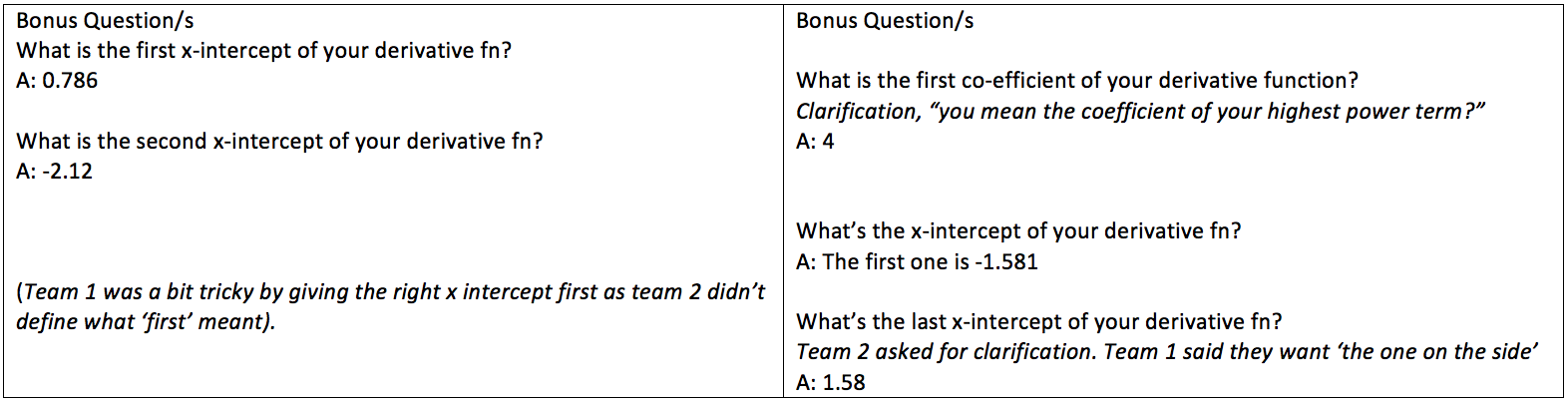 It can be seen that the team on the left got the hint and jumped into asking for the zeroes of the derivative to find the turning point. The team on the right tried a similar thing, but only on their second turn, and they were playing copy-cat so didn’t quite know why they were asking the question. Results? Here’s what was produced…
It can be seen that the team on the left got the hint and jumped into asking for the zeroes of the derivative to find the turning point. The team on the right tried a similar thing, but only on their second turn, and they were playing copy-cat so didn’t quite know why they were asking the question. Results? Here’s what was produced…
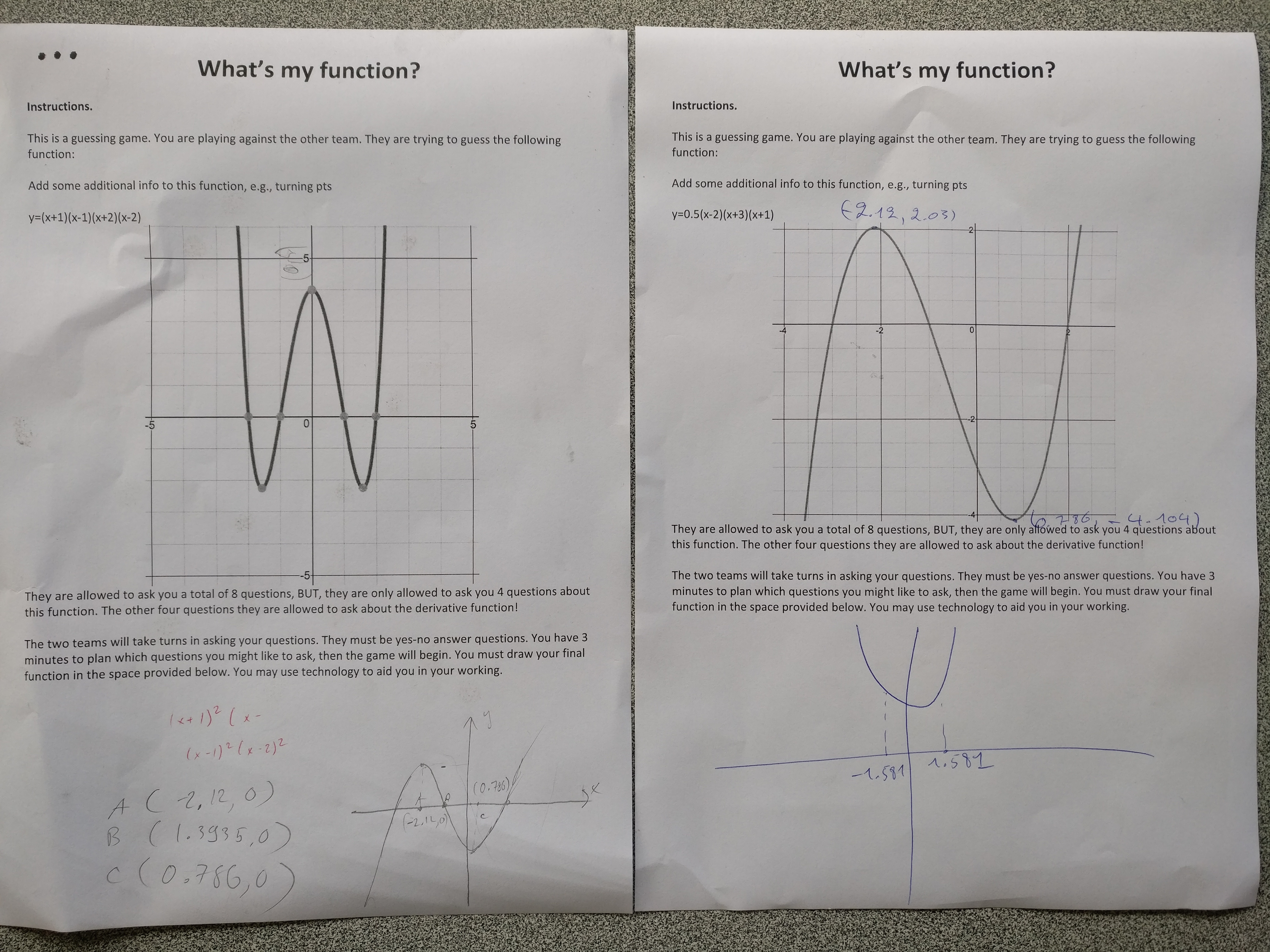 By this time we were up against the end of the period so I took a photo of the fns to prompt discussion at the start of the following lesson.
By this time we were up against the end of the period so I took a photo of the fns to prompt discussion at the start of the following lesson.
In the following period, I asked one basic question of the team who drew the fn on the bottom right: “Why did the other team ask for the zeroes of your derivative function?”. A long discussion ensued but we managed to boil it down to the following spaced repetition card…
 I guess I could have just told them that at the start, but that would have taken out the joy and excitement of making the connection themselves*. We’ll see if it helped it stick… term break starts tomorrow!
I guess I could have just told them that at the start, but that would have taken out the joy and excitement of making the connection themselves*. We’ll see if it helped it stick… term break starts tomorrow!
*Many readers will already be familiar with Paul Lockhart’s ‘A Mathematician’s Lament‘ on this point.
